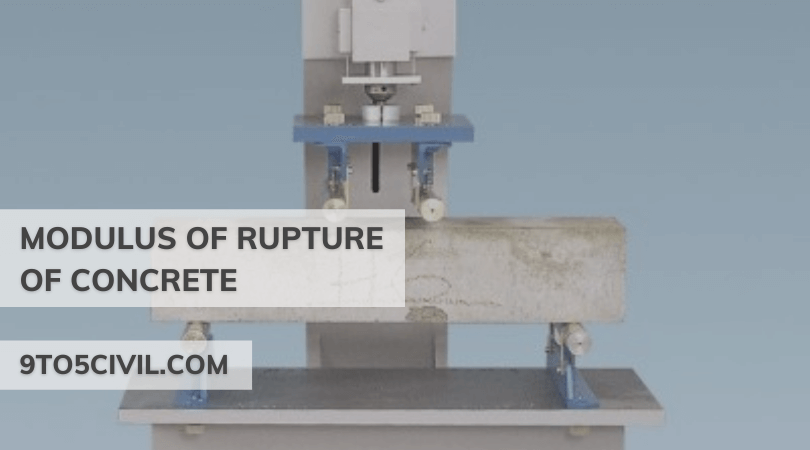
What Is Modulus of Rupture of Concrete? | What Is Modulus Rapture? | Standard Test | Methods of Modulus of Rupture of a Beam | Calculation of Modulus of Rupture

What Is Modulus of Rupture of Concrete?

The modulus rapture of a concrete formula is defined as measuring the tensile strength contained in concrete beams or slabs. Flexural strength, defined as the modulus of rupture, or band strength, or the strength of transverse rupture, is defined as the material stress used in a building. Flexural strength is tested before any material is used.

Calculation of modulus of rupture as a member of construction is considered important for the following reasons. Calculation of modulus of rupture helps in maintaining the construction of structural elements like beams, cantilevers, shafts, etc.

Modulus rapture provides a parameter for the structure of a dynamic building. It is a predictive tool for resistance and durability to the structure of construction.

Also Read : What Is Tension? | What Is Compression? | Difference Between Compression and Tension

What Is Modulus Rapture?

Modulus Rupture indicates the bending strength of the material used in construction and its suitability for use in construction.

The strength of the product bonding system can be known by modulus rapture. And is an important factor for building quality control and development.

Modulus rapture is the material’s own physical property. The flexure test is tested before it is used for material stress.

The most common way to obtain the flexural strength of a material is to use a three-point flexural testing technique. It also measures the flexural strength of the material through the transverse bending test.

Modulus of Rupture is defined as the final strength of the beam, which is related to the failure of the beam by the flexibility equal to the moment of bending divided in the cross-section of the beam.

Also Read : What Is Floating Slab? | Purpose of Floating Slab | Construction of Floating Slab | Advantage & Disadvantage of Floating Slab

Importance of Modulus of Rupture of Concrete Beam

1. It is a technique for estimating both the resistance and durability of materials.
2. It helps in designing the structural elements of the building like beams, cantilevers, shafts, etc.
3. Assists in the study of its use according to the materials and their properties
4. Provides parameters for the development and quality of strong building materials.
5. Flexural strength helps determine the design and quality of structures for constructions.
6. The calculation of the modulus of rupture is considered crucial for the future of the building.

Also Read : What Is Bridge Abutment? | Uses of Bridge Abutment | Components of Bridge Abutment | Type of Abutment

What Is Flexural Modulus?

Flexural modulus or bending modulus is the intensive property of the material in mechanics. The tendency of the material to resist flexural deformation and bending moment is estimated.

The stress-strain curve is determined by a flexural test (such as the ASTM D790). And this curve uses units of force per unit area.

Flexural modulus is defined using a 3-point curvature test that shows a linear stress-strain response.

Ideally, the flexural modulus of elasticity is equivalent to the compressive modulus or tensile modulus (Young’s modulus) of elasticity. The values of each of these materials may be different, especially for materials such as polymers which are viscoelastic (time-dependent) materials.

The flexural modulus contains both the tensile and compressive strains in the curve patterns of the compressive and tensile strain.

There are usually different compressive and tensile modules for the same material as polymers.

Also Read : What Is False Work? | Types of Falsework | Causes of Falsework Failures

What Is Flexural Stress?

Stress caused by the bending moment generated in a structural member is known as Flexural Stress. Flexural stress usually occurs in two ways.

In one case, there is stress on the supported beam. And in the second case, there is stress in the cantilever beam. Flexural Stress works differently for simply supported beams.

The upper surface of any beam resists compression stress. And the lower surface of the beam resists tension stress flexural stress.

The formula of flexural stress,

σ =MC / I. 

M = Bending moment

C = Distance from Neutral Axis

I = moment of inertia

Also Read : What Are Bond Breakers? | Materials Used in Bond Breaker | Type of Concrete Bond Breaker | Advantage of Bond Breaker

Standard Test Methods of Modulus of Rupture of a Beam

To test the flexural strength of a given concrete beam, the span length of that beam should be at least three times its depth.

The flexural strength of the material is expressed as modulus of rupture (MR) as psi (MPa) unit. The flexural strength of a concrete beam is obtained by two standard testing methods.

1. Center Point Loading Test

In this method, the entire load is given in the center of the span length of the beam.

In this test method, the flexural strength or modulus of rupture value is higher than the third point loading test.

The maximum stress produced by this test is only in the center of the beam.

2. Third Point Loading Test

In the process of the third point loading test, the half load is applied on every third part of the total span of the concrete beam.

The value of the modulus of rupture found in the third point loading test is less than the value found in the center point loading test.

In this test method, maximum tension is generated in the center of one-third of the span of the beam.

The flexural modulus of rupture is 10% to 20% of the compressive strength of concrete depending on the type, volume, and size, of coarse aggregate used in the concrete beam.

However, given the laboratory tests, the option of specific materials for the given material and mix design is obtained.

The value of the modulus of rupture of the material determined by the third point loading is less than the value of the modulus of rupture determined by the center point loading.

Also Read : What Is the Well Point System? | Types of Well Point System | Advantages & Disadvantages of Well Point System

Calculation of Modulus of Rupture

The formula for modulus of rupture is different for different types of loading systems applied to the concrete.

1. The Calculation for the Given Load for a Three-Point Bending Setup on a Rectangular Sample

Formula = 1.

Modulus of Rupture σ= (3FL)/(2bd²)

• F = The load (force) at the fracture point (N)
• L = The length of the member
• b = Width of the member
• d = thickness of the  member

2. For a Rectangular Sample, Under Load in a Four-Point Bending Setup, Where the Loading Span Is One-Third of the Supported Member

Formula = 2.

Modulus of Rupture = (FL)/(bd²)

• F = The load (force) at the fracture point (N)
• L = The length of the member
• b = Width of the member
• d = thickness of the  member

Also Read : Light weight Concrete | Properties of Lightweight Concrete | Type of Lightweight Concrete | Uses of Lightweight Concrete

3. For Three-Point Bending Setup and Four-Point Bending Setup, If the Loading Span Is 1/2 of the Support Member

Formula = 3.

Modulus of Rupture = (3FL)/(4bd²)

• F = The load (force) at the fracture point (N)
• L = The length of the member
• b = Width of the member
• d = thickness of the  member

4. Where the Loading Span Is Neither 1/3 nor 1/2 on the Support Member for the Four-Point Bending Setup

Formula = 4.

Modulus of Rupture = (3F[L-L_i])/(2bd²)

• F = The load (force) at the fracture point (N)
• L = The length of the member
• b = Width of the member
• d = thickness of the  member
• L_i = the length of the loading (inner) span

Also Read : What Is Isometric Projection? | Principle of Isometric Projections | Isometric Scale | What Is Isometric Drawing? | Methods of Isometric Drawing